It’s pretty uncommon to randomly run across someone who was born on the same day of the year you were. But the birthday paradox proves that it is not nearly as rare as you think it is.
What is the birthday paradox?
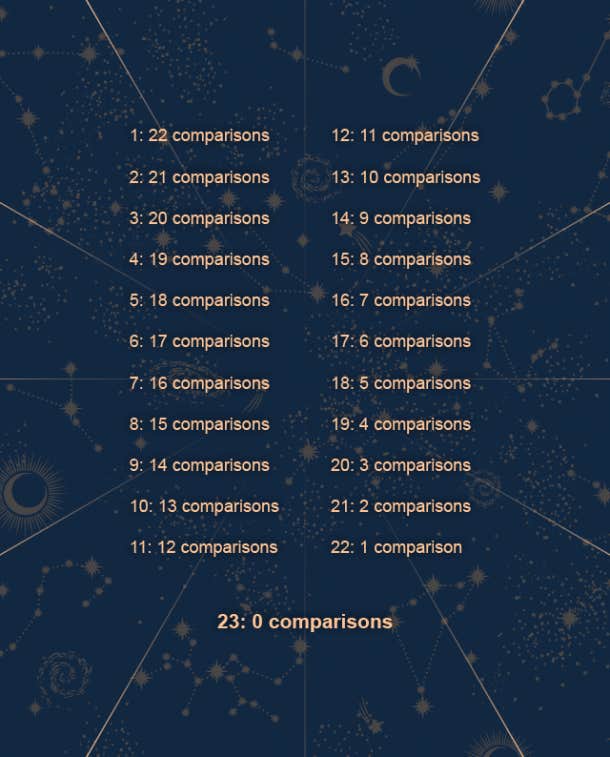
The birthday paradox, otherwise known as the birthday problem, theorizes that if you are in a group of 23 people, there is a 50/50 chance you will find a birthday match. RELATED: Why You Keep Seeing Your Birthdate Everywhere The theory has been labeled a paradox because, with only 23 people in the room, you have just 22 comparisons to make or 22 chances that two people have a shared birthday. At first glance, it seems is that the number of people in the sample size is much too small to find two people who are a birthday match. However, when all 23 of the birthdays are compared to one another, there are 253 comparisons, thus a much higher likelihood of two people sharing a birthday. How are there 253 comparisons, you ask? Well, starting with the first person, you compare their birthday to the other 22 people in the group of 23. For the second person, they have already been compared to the first person, so you just compare them to the remaining 21 people. Once you get to person number 3, they have already been compared to numbers 1 and 2, so they only need to be compared to 20 people. Each time you move on to the next person, the number of comparisons drops by one, as seen below:
RELATED: How To Tell If Someone Is Your Soulmate Using Simple Math By the time you get to the 23rd person, all of the comparisons have been made, so there are no more people to compare them to. If you do the math, you will find that you have compared a total of 253 pairs of birthdays: 22 + 21 + 20 + 19 + 18 + 17 + 16 + 15 + 14 + 13 + 12 + 11 + 10 + 9 + 8 + 7 + 6 + 5 + 4 + 3 + 2 + 1 = 253 This is where the math can get a little more complex. When coming up with the probability of a person’s birthday matching someone in the group, it’s easier to look at how likely it is that people have different birthdays. Let’s simplify by just looking at two people, one comparison. A person’s birthday is one out of 365 days, except on leap years when it is one out of 366 days. Because we’re looking at the likelihood of not sharing a birthday, you divide 364 (the number of days that are not your birthday) by 365 and determine that there is a 99.726027% chance of there being no match. 364 / 365 = 99.726027% From our earlier calculations, we know that a group of 23 people has 253 comparisons. Now, we calculate the probability of people in that group not sharing a birthday as follows: 99.726027% is multiplied by itself 253 times (99.726027253) to come up with a 49.952% probability that there are no matches. That means the possibility of finding a match among the 23 people is 50.048%! To test this “paradox,” grab 10 to 12 groups of 23 people each and do a birthday comparison. Your results should end up at around 50%. So, rest assured that it’s totally likely to find your birthday twin when you least expect it. RELATED: What Science Says About Your Chances Of Having A Doppelgänger Or ‘Twin Stranger’ NyRee Ausler is a writer from Seattle, Washington, and author of seven books. She covers lifestyle, entertainment and news, and self-focused content, as well as navigating the workplace and social issues.